|
|
|
|
| Whiteboard |
|
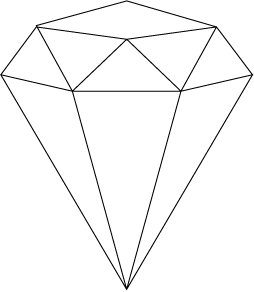
| A classical gem cut for a diamond is shown. Note that light is intended to enter the gem from the planar surfaces (called facets) on top, and the pointed base of the gem is encased in a setting and out of sight. This design takes advantage of Total Internal Refraction (TIR). |
| Q1: Using a diagram,
derive the formula for calculating the critical angle given index
of refraction of a substance in air, which is 1.00.
Q2: If a diamond has an index of refraction n = 2.42, what is the critical angle for a diamond in air? Q3: Why are the top facets of this diamond at such a steep angle and the lower facets at such a shallow angle to a ray of light entering vertically from above? Q4: Why does a perrfectly clear diamond appear blue and not red in sunlight? |
| References |
|
|
|
|