Q1: Using
a diagram, derive the formula for calculating the critical angle given
index of refraction of a substance in air, which is 1.00.
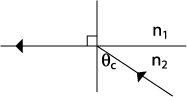 |
n1sinq1 = n2sinq2
n1sin90° = n2sinqc
assume n1 = 1.00 (air)
(1)(1) = n2sinqc
sinqc = 1/n
|
Q2: If a diamond
has an index of refraction n = 2.42, what is the critical angle
for a diamond in air?
sinqc = 1/ndiamond
sinqc = 1/2.42
qc = 24.4°
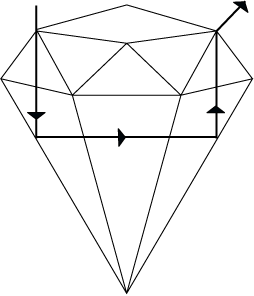
Q3: Why are the top
facets of this diamond at such a steep angle and the lower facets
at such a shallow angle to a ray of light entering vertically from
above?
| The idea is to readily admit and let light
escape from the top qi
< qc; qr
< qc but have TIR
at all surfaces below the top so light reflects within the diamond
qi = qr>
qc until it escapes out
the top. |
 |
Q4: Why
does a perfectly clear diamond appear blue and not red in sunlight?
Blue is deviated most and therefore is more easily reflected
back out whereas red is reflected least and has more trouble being
reflected out and is more likely absorbed.
|

