|
|
|
|
| Whiteboard |
|
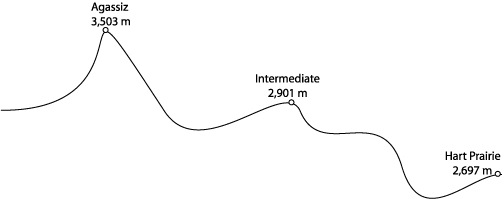
At an unnamed Arizona ski resort, a 60.0 kg skier starts from rest at a point called Agassiz at an elevation of approximately 11,500 ft down a slope to an intermediate point of elevation 9,519 ft. During this section of the run, the skier loses approximately 400 kJ of energy to air resistance and snow friction. The skier gains 50 kJ by poling and other body movement.
|
|
Q1: What is the skier's speed at the intermediate point? During the second half of the total run, the skier moves through a series of side trails and down a slope called Hart Prairie to a final elevation of 8,850 ft. During stage two the skier loses 170 kJ to frictional/air resistance, but gains an additional 50 kJ of energy due to body work. Q2: What is the skier's final velocity (Vf) at the foot of the Hart Prairie run? Q3: Please draw a crude sketch calculating kinetic energy (KE), gravitational potential energy (GPE), and total energy (TE) at the three points labeled Agassiz (start), Intermediate, and Hart Prairie (final). Use SI (m, k and s) units throughout. Remember [1.0 m = 3.281 ft] Advanced Question: Near the very foot of Hart Prairie run there is a small circular 'speed bump' hill of radius 5.0 m. At what minimum Vf will the skier become airborne? Does this particular skier become airborne? (Draw a FBD) |
| References |
| MacIsaac, after Reif F (1995). Understanding Basic Mechanics. Wiley: NY. W180. |
|
|
|
|